Validation of liquid chromatography mass spectrometry (LC-MS) methods
9.4. Estimation of LoQ
Different approaches to estimate
http://www.uttv.ee/naita?id=23684
https://www.youtube.com/watch?v=G8avqFKe0ds
Approaches to estimate LoQ can generally be divided into two groups: (a) based on the estimation of and (or uncertainty) at different concentration levels, or (b) based on the similar approaches that are used to estimate . However, in the case when a specific guideline must be followed, then the LoQ must be estimated by following the guideline.
First let’s discuss the approaches to determine LoQ by estimating precision and trueness of the method at multiple concentration levels. LoQ can then be taken as the lowest concentration where these parameters are fit for purpose (set by e.g. the analyst or client) or meet the requirements of the necessary standards or guidelines. For example, requires that the intensities at LoQ must have precision of 20% at most and trueness of ±20% (i.e. the average result should stay between 80-120% of the reference value), and SANTE/SANCO requires that mean (recovery is meant here as trueness) is in range of 70–120% and relative standard deviation (which indicates precision) of at most 20%.
See section 4, 5 and 6 for estimating trueness and precision and section 7 for further information about uncertainty.
Moreover, it has been suggested that the LoQ should be found by expressing the uncertainty of the measurement as a function of concentration and comparing the results to the uncertainty levels demanded of that method.
Although the definitions of LoD and LoQ differ significantly, the second group of approaches to estimate LoQ use similar approaches that are used for estimating LoD. However, in case of LoQ, a greater multiplication coefficient k (used in place of Student’s Coefficient) in the equation is used or other higher demands are set on the same parameters. For example, S/N value of at least 10 is required at the LoQ concentration level.
The following equation can be used to estimate LoQ:
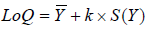(Eq 1)
Here the same variables can be used as with LoD (see Table 1) and the same experimental design (e.g. concentration levels and number of replicate measurements) is required. However, the coefficient k is required to have values of 5, 6 or 10 (depending on standard or guideline that is being followed). In case of the ICH approach (using the calibration function to estimate the standard deviation) the following equation can be used []:
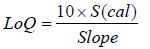(Eq 2)
Here again all the variables can be taken from the same datasets for both LoD and LoQ. Visual evaluation can also be used to estimate LoQ: the LoQ is taken as the lowest concentration level where the analyte can be quantified with acceptable level of precision and trueness. It has also been suggested that LoQ can be found by simply multiplying LoD by 2.
LoQ is determined in most approaches from the same data as LoD or is based on LoD and therefore in principle the same issues occur. However, these approaches can over- or underestimate the LoQ because they do not look at the trueness and precision values at specific concentrations. It is assumed that the properties of the analytical methods are relatively similar and above the defined point over LoD, the of the results will be fit for purpose.
We recommend using the first group of approaches: precision and trueness should be estimated at different concentration levels and the lowest level where both parameters are in agreement with the demanded value is taken as LoQ. Although labour-intensive, this approach estimates LoQ by its exact definition.
However, if LoQ is not critically important, then using the approach given by ICH (Eq 2, using the standard deviation of residuals) is suggested.
It has also been stated that the LoQ value can be taken as the lowest concentration level used for calibration. This can be convenient if the LoQ of the analytical method value is significantly below the . In this case extra measurements do not have to be made at lower concentrations specifically for estimating LoQ. However, it should still be shown, that the method is capable of fit-for-purpose trueness and precision at the lowest concentration level.
About some important aspects of LoQ
The aspects of LoD and LoQ estimation are often similar.
LoQ is also used for two purposes: (a) to determine whether the measured concentration of the sample is in the range that allows it to be quantified with fit for purpose accuracy with the given analytical method, and (b) to characterize the analytical method.
When estimating LoQ, the data used to estimate it should be in the range of LoQ. If a calibration function is used to estimate it, then and have similar effects on the LoQ estimation as on the LoD estimation.
The samples used to estimate LoQ should be matrix matched.
As LoD, trueness and precision vary between days (and between measurement series due to random variance of results), LoQ values also have to be estimated on different days.
Due to the variance between days, we recommend that LoQ should be determined 5 times over a longer period and the most conservative result should be stated as the methods’ performance level to increase its reliability. Moreover, the methods’ performance at the LoQ level can be monitored with regular analysis of samples (either real contaminated samples or spiked blank samples) with concentrations close to LoQ.
The exact way of determining LoQ should be specified as with LoD due to the differences of the results when different approaches are used. The difference in LoQ estimates can, however, also come from the fact that different precision and trueness limits have been set.
Calculating LoQ *Note 1
http://www.uttv.ee/naita?id=24441
https://www.youtube.com/watch?v=DXiGL72twow
***
*Note 1: NB! In this video we do not consider the statistical significance of intercept (intercept < 2 times the standard deviation of intercept). Although this is a simplification, the difference between the results when intercept is or is not set to 0 is not significant. i.e. the variance between the LoQ values obtained on different days is significantly larger than the difference caused by the change of the intercept value.
