Estimation of measurement uncertainty in chemical analysis

Self-test 9 A

Here you can test your understanding of the ISO GUM modeling approach and your ability to apply it in practice.

The acidity (expressed as concentration of H+ ions in mol/l) of an acidic liquid (sample) is measured by titration with KOH solution using phenolphthalein as indicator. 10 ml aliquots of the liquid are titrated. The liquid is measured using a 10 ml pipette. The uncertainty of pipetting (taking into account all uncertainty components) is ± 0.06 ml. Titration was carried out 5 times and the consumed titrant volumes were (all in ml): 12.35, 12.54, 12.21, 12.49 and 12.29. In this case it is safe to assume that the uncertainty of titrant volume originates from two sources: repeatability and uncertainty due to possible systematic effect of finding the end-point. The latter is estimated as ± 0.15 ml. Titrant concentration has been determined in the laboratory earlier and it is (0.1230 ± 0.0015) mol/l, k =2, norm.

Find the acidity of the investigated liquid and the standard uncertainty. 

Uncertainty components of c(H+) are: pipetted volume of the sample (u(Vsample)), volume of the titrant (VKOH) and concentration of the titrant (cKOH). Mathematical model: 9-1-1.png

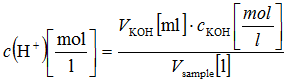
Uncertainty of pipetting has only one component that includes all uncertainty contributors and as it has been presented as ± 0.06 ml without further information on its distribution. It is therefore the safest to assume that it is rectangularly distributed.

Uncertainty of titrant volume has two components: uncertainty due to repeatability and uncertainty due to possible systematic effect of finding the end-point. Repeatability can be found as the standard deviation of the mean from the titration results. End-point is estimated as ± 0.15 ml and here it is the safest to assume rectangular distribution.

Standard uncertainty can be found from expanded uncertainty by dividing the latter by coverage factor. In order to find standard uncertainty from the uncertainty with rectangular distribution it is divided by 9-1-2.png.

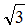
In case of model that includes only multiplications and divisions is the correct way is to calculate the relative (unitless) standard uncertainties and then combine those according to the following equation: 9-1-3.png
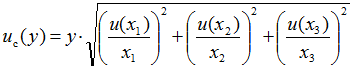
The acidity of the investigated liquid is:

The combined standard uncertainty of the found acidity is:


 

Previous
Next
Accept Cookies